Then T is said to be an affine transformation if:
- T maps vectors to vectors and points to points
- T is a linear transformation on the vectors
-
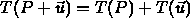
T preserves affine combinations on the points:
![]()
where ![]()
- Affine transformations map lines to lines:
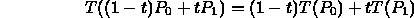
- Affine transformations preserve ratios of distance
along a line
(converse is also true: preserves ratios of such distances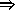 affine).
affine).
- Which is a larger class of transformations: Affine or Linear?
- T is linear if T(aA+bB)=aT(A)+bT(B) for all a,b.
- T is affine if T(aA+bB)=aT(A)+bT(B) if a+b=1.
- If T is linear, then T(aA+bB)=aT(A)+bT(B) when a+b=1 so T is clearly affine.
- But if T is affine and
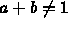 then T(aA+bB)
might not equal aT(A)+bT(B).
then T(aA+bB)
might not equal aT(A)+bT(B).
Thus, it is possible for T to be affine but not linear.
- Therefore, affine transformations are a superclass of linear transformations (surprise!)
- An alternate way to think of this is that the behaviour of
linear transformations is restricted for a wider range
of values.
Consider T(aA+bB)
- Linear transformations have their behaviour specified for all values of a,b.
- Affine transformations have their behaviour specified only when a+b=1. They can behave differently for all other values of a+b.
- Most of the transformations we consider will be linear.
- Translation is the only non-linear (but affine) transformation we'll see, and we'll ``bypass'' its non-linear behavior.
Proof: Let ![]() and
and ![]() .
.
Suppose ![]() , which implies
, which implies
![]() by linearity.
by linearity.
Then
![]()
and
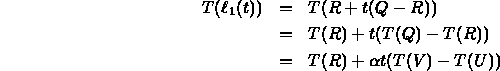
and we see that the images of both lines are parallel.
- There exists points Q and R such that
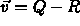 .
.
- Define
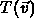 to be T(Q)-T(R).
to be T(Q)-T(R).
Note that Q and R are not unique.
The definition works for ![]() :
:
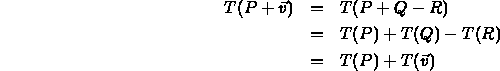
This can now be used to show that the definition is
well defined.
If Q-R=B-C then

- Let
-
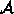 and
and  be affine spaces.
be affine spaces.
- Let
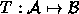 be an affine transformation.
be an affine transformation.
- Let
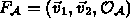 be a frame
for
be a frame
for 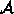 .
.
- Let
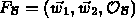 be a frame
for
be a frame
for  .
.
- Let P be a point in
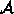 whose coordinates relative
whose coordinates relative
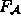 are
are  .
.
- (
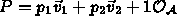 )
)
- Let
- Question:
- What are the coordinates
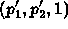 of T(P) relative to
of T(P) relative to  ?
?
![]()
If
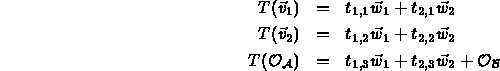
then we can find ![]() by substitution and
gathering like terms.
by substitution and
gathering like terms.  \
\